

The wavelength λ of a musical note with a frequency f n can be calculated as The frequency 440.2542274 Hz is 1 cent above A4:

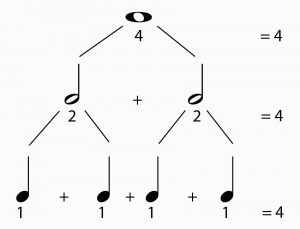
The formula above gives n b = 100.0008857 ≈ 100 cents To calculate the bias from a note in cents n b from known frequency f n, we will use the following formula:įor example, A4♯/B4♭ has the frequency of 466.164 Hz. In other words, one cent is 1/100 of a semitone. The cent is a dimensionless logarithmic unit of measurement of musical intervals defined as the ratio of two frequencies f 1 and f 2, which is equal to:ġ00 cents make one semitone of the equal-tempered scale. Note that the ratio of the frequencies of the two notes in any semitone interval a is equal to the twelfth root of 2: There are 88 notes in the standard piano keyboard, from note A0 (sub-contra octave), which is the piano note 0 with a frequency of 27.500 Hz, to note C8, which is the piano note 88 with a frequency of 4186.009 Hz. To calculate frequencies of musical notes from known standard (88-key) piano note number n p and the standard pitch A440, and vice versa, we can use the following formulas: There are 128 notes in the MIDI standard (0 to 127), from the note C–1 (minus 1), which is the MIDI note 0 with a frequency of 8.176 Hz, to the note G9, which is the MIDI note 127 with a frequency of 13289.750 Hz. To calculate frequencies of musical notes from known MIDI note number n m and the standard pitch A440, and vice versa, we can use these formulas: The formula above can be modified to calculate the number n of semitones away from the standard pitch f 0: N is the number of semitones (half steps) from the standard pitch n > 0 for notes higher than the standard pitch, and n < 0 for notes lower than the standard pitch. It is usually a standard (also called concert) pitch of 440 Hz, which is called A440 or note A in the one-line (or fourth) octave (A4) But from now on, we will be notating most of our musical examples fully, using the correct staff symbols to show the lengths of the notes.The basic formula to calculate frequencies of musical notes of the equal-tempered scale:į n is the frequency of the note, which is n semitones (or half steps) away from the standard pitch A440 į 0 is the frequency of a fixed note, which is used as a standard for tuning. We will still use it where timing is not important, such as discussing the notes of a single chord. This makes it perfect to indicate where no attempt at showing note lengths has been made. In fact, the simple black notehead is not a proper staff notation symbol at all. Staff notation has a range of symbols for showing note length, using a combination of hollow and solid noteheads, stems, and flags. And yet they look the same if they are notated without indicating their note lengths.Ĭlearly, the shorthand notation we have used so far in these tutorials is not adequate for accurately describing a piece of music. You will agree the variations sound very different from the original melody, as if they belong to a different song. The other two contain the same notes, in the same sequence, but with different lengths. The original is easily recognized as Mary Had A Little Lamb. Shown below are three variations on a melody. What may not be so obvious is how dramatically the lengths of the notes can affect the melody of a piece of music. The melodic notes are very short and fast, while the harmonic (chord) notes are longer and fewer. Consider the passage below, composed by Rimsky-Korsakov. It is obvious that in music, notes of many different lengths are played.


 0 kommentar(er)
0 kommentar(er)
